Ver no TensorFlow.org Ver no TensorFlow.org |  Executar no Google Colab Executar no Google Colab |  Ver fonte no GitHub Ver fonte no GitHub |  Baixar caderno Baixar caderno |
Visão geral
Este bloco de notas demonstrará como usar o Conditional Graident Optimizer do pacote de complementos.
ConditionalGradient
Restringir os parâmetros de uma rede neural mostrou ser benéfico no treinamento por causa dos efeitos de regularização subjacentes. Freqüentemente, os parâmetros são restringidos por meio de uma penalidade suave (que nunca garante a satisfação da restrição) ou por meio de uma operação de projeção (que é computacionalmente cara). O otimizador de gradiente condicional (CG), por outro lado, impõe as restrições estritamente sem a necessidade de uma etapa de projeção dispendiosa. Ele funciona minimizando uma aproximação linear do objetivo dentro do conjunto de restrições. Neste bloco de notas, você demonstra a aplicação da restrição da norma Frobenius por meio do otimizador CG no conjunto de dados MNIST. CG agora está disponível como uma API tensorflow. Mais detalhes sobre o otimizador estão disponíveis em https://arxiv.org/pdf/1803.06453.pdf
Configurar
pip install -q -U tensorflow-addons
import tensorflow as tf
import tensorflow_addons as tfa
from matplotlib import pyplot as plt
# Hyperparameters
batch_size=64
epochs=10
Construir o modelo
model_1 = tf.keras.Sequential([
tf.keras.layers.Dense(64, input_shape=(784,), activation='relu', name='dense_1'),
tf.keras.layers.Dense(64, activation='relu', name='dense_2'),
tf.keras.layers.Dense(10, activation='softmax', name='predictions'),
])
Prepare os dados
# Load MNIST dataset as NumPy arrays
dataset = {}
num_validation = 10000
(x_train, y_train), (x_test, y_test) = tf.keras.datasets.mnist.load_data()
# Preprocess the data
x_train = x_train.reshape(-1, 784).astype('float32') / 255
x_test = x_test.reshape(-1, 784).astype('float32') / 255
Definir uma função de retorno de chamada personalizada
def frobenius_norm(m):
"""This function is to calculate the frobenius norm of the matrix of all
layer's weight.
Args:
m: is a list of weights param for each layers.
"""
total_reduce_sum = 0
for i in range(len(m)):
total_reduce_sum = total_reduce_sum + tf.math.reduce_sum(m[i]**2)
norm = total_reduce_sum**0.5
return norm
CG_frobenius_norm_of_weight = []
CG_get_weight_norm = tf.keras.callbacks.LambdaCallback(
on_epoch_end=lambda batch, logs: CG_frobenius_norm_of_weight.append(
frobenius_norm(model_1.trainable_weights).numpy()))
Treine e avalie: usando CG como otimizador
Basta substituir os otimizadores keras típicos pelo novo otimizador tfa
# Compile the model
model_1.compile(
optimizer=tfa.optimizers.ConditionalGradient(
learning_rate=0.99949, lambda_=203), # Utilize TFA optimizer
loss=tf.keras.losses.SparseCategoricalCrossentropy(),
metrics=['accuracy'])
history_cg = model_1.fit(
x_train,
y_train,
batch_size=batch_size,
validation_data=(x_test, y_test),
epochs=epochs,
callbacks=[CG_get_weight_norm])
Epoch 1/10 938/938 [==============================] - 4s 3ms/step - loss: 0.6034 - accuracy: 0.8162 - val_loss: 0.2282 - val_accuracy: 0.9313 Epoch 2/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1968 - accuracy: 0.9411 - val_loss: 0.1865 - val_accuracy: 0.9411 Epoch 3/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1502 - accuracy: 0.9552 - val_loss: 0.1356 - val_accuracy: 0.9590 Epoch 4/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1349 - accuracy: 0.9598 - val_loss: 0.1084 - val_accuracy: 0.9679 Epoch 5/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1261 - accuracy: 0.9609 - val_loss: 0.1162 - val_accuracy: 0.9648 Epoch 6/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1119 - accuracy: 0.9662 - val_loss: 0.1277 - val_accuracy: 0.9567 Epoch 7/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1096 - accuracy: 0.9671 - val_loss: 0.1009 - val_accuracy: 0.9685 Epoch 8/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1045 - accuracy: 0.9687 - val_loss: 0.1015 - val_accuracy: 0.9698 Epoch 9/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1011 - accuracy: 0.9688 - val_loss: 0.1180 - val_accuracy: 0.9627 Epoch 10/10 938/938 [==============================] - 3s 3ms/step - loss: 0.1029 - accuracy: 0.9689 - val_loss: 0.1590 - val_accuracy: 0.9516
Treine e avalie: usando SGD como otimizador
model_2 = tf.keras.Sequential([
tf.keras.layers.Dense(64, input_shape=(784,), activation='relu', name='dense_1'),
tf.keras.layers.Dense(64, activation='relu', name='dense_2'),
tf.keras.layers.Dense(10, activation='softmax', name='predictions'),
])
SGD_frobenius_norm_of_weight = []
SGD_get_weight_norm = tf.keras.callbacks.LambdaCallback(
on_epoch_end=lambda batch, logs: SGD_frobenius_norm_of_weight.append(
frobenius_norm(model_2.trainable_weights).numpy()))
# Compile the model
model_2.compile(
optimizer=tf.keras.optimizers.SGD(0.01), # Utilize SGD optimizer
loss=tf.keras.losses.SparseCategoricalCrossentropy(),
metrics=['accuracy'])
history_sgd = model_2.fit(
x_train,
y_train,
batch_size=batch_size,
validation_data=(x_test, y_test),
epochs=epochs,
callbacks=[SGD_get_weight_norm])
Epoch 1/10 938/938 [==============================] - 3s 3ms/step - loss: 1.4885 - accuracy: 0.5945 - val_loss: 0.4230 - val_accuracy: 0.8838 Epoch 2/10 938/938 [==============================] - 2s 2ms/step - loss: 0.4087 - accuracy: 0.8875 - val_loss: 0.3222 - val_accuracy: 0.9073 Epoch 3/10 938/938 [==============================] - 2s 2ms/step - loss: 0.3267 - accuracy: 0.9075 - val_loss: 0.2867 - val_accuracy: 0.9178 Epoch 4/10 938/938 [==============================] - 2s 2ms/step - loss: 0.2903 - accuracy: 0.9186 - val_loss: 0.2605 - val_accuracy: 0.9259 Epoch 5/10 938/938 [==============================] - 2s 2ms/step - loss: 0.2691 - accuracy: 0.9233 - val_loss: 0.2468 - val_accuracy: 0.9292 Epoch 6/10 938/938 [==============================] - 2s 2ms/step - loss: 0.2466 - accuracy: 0.9291 - val_loss: 0.2265 - val_accuracy: 0.9352 Epoch 7/10 938/938 [==============================] - 2s 2ms/step - loss: 0.2210 - accuracy: 0.9370 - val_loss: 0.2106 - val_accuracy: 0.9404 Epoch 8/10 938/938 [==============================] - 2s 2ms/step - loss: 0.2137 - accuracy: 0.9387 - val_loss: 0.2029 - val_accuracy: 0.9424 Epoch 9/10 938/938 [==============================] - 2s 2ms/step - loss: 0.1996 - accuracy: 0.9429 - val_loss: 0.1937 - val_accuracy: 0.9441 Epoch 10/10 938/938 [==============================] - 2s 2ms/step - loss: 0.1925 - accuracy: 0.9450 - val_loss: 0.1831 - val_accuracy: 0.9469
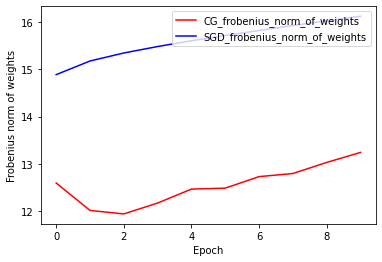
Norma de Pesos Frobenius: CG vs SGD
A implementação atual do otimizador CG é baseada na norma Frobenius, considerando a norma Frobenius como regularizador na função de destino. Portanto, você compara o efeito regularizado do CG com o otimizador SGD, que não impôs o regularizador Frobenius Norm.
plt.plot(
CG_frobenius_norm_of_weight,
color='r',
label='CG_frobenius_norm_of_weights')
plt.plot(
SGD_frobenius_norm_of_weight,
color='b',
label='SGD_frobenius_norm_of_weights')
plt.xlabel('Epoch')
plt.ylabel('Frobenius norm of weights')
plt.legend(loc=1)
<matplotlib.legend.Legend at 0x7fada7ab12e8>
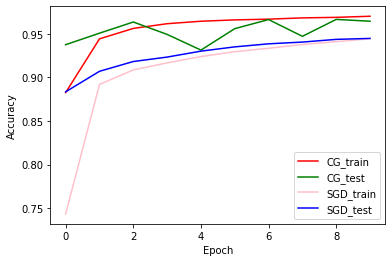
Precisão de treinamento e validação: CG vs SGD
plt.plot(history_cg.history['accuracy'], color='r', label='CG_train')
plt.plot(history_cg.history['val_accuracy'], color='g', label='CG_test')
plt.plot(history_sgd.history['accuracy'], color='pink', label='SGD_train')
plt.plot(history_sgd.history['val_accuracy'], color='b', label='SGD_test')
plt.xlabel('Epoch')
plt.ylabel('Accuracy')
plt.legend(loc=4)
<matplotlib.legend.Legend at 0x7fada7983e80>